Strange dances of electrons in periodic landscapes
Single particles can move in very strange ways if exposed to
periodically structured environments. Think of an electron moving
in a crystal. The crystal consists of atoms situated on a periodic
lattice. Here the electron will typically perform chaotic motion,
where the path of the electron looks seemingly random and
irregular. Naively one would expect that the average distance an
electron can pass per time step increases if, say, one increases the
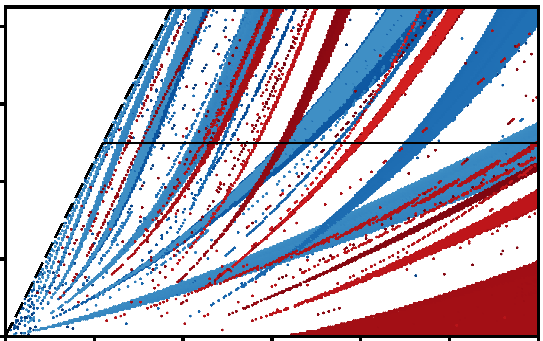
distance between two nearby atoms. In a recent Letter Klages et
al. have found that this is not the case. Rather, the spreading of
particles in a periodic lattice depends in a highly non-trivial way on
the variation of system parameters. This phenomenon is microscopically
explained in terms of periodic orbits: For certain parameter values
the particles find channels along the lattice in which they can wiggle
around fast in one direction while at other values these channels
disappear. This finding may have important applications to electronic
transport in graphene-like structures, a novel material that became
very popular after being valued by a Nobel prize in 2010.
R. Klages, S.S.G. Gallegos, M. Sarvilahti, J. Solanpää, E. Räsänen, Normal and anomalous diffusion in soft Lorentz gases, Physical Review Letters 122, 064102 (2019)